Understanding Resistivity

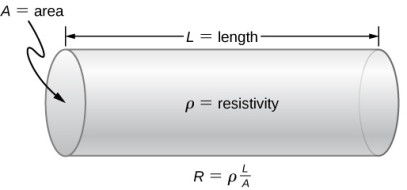
Resistivity (\( \rho \)) quantifies how strongly a material opposes the flow of electric current. It is
calculated using the formula:

\[\rho = \frac{R \cdot A}{L}\]

Where:

• \( \rho \): Resistivity (Ω·m)
• \( R \): Resistance (Ω)
• \( A \): Cross-sectional area (m²)
• \( L \): Length of the material (m)

Example Calculations

Example 1

Given:

• \( R = 10 \, \Omega \)
• \( A = 0.5 \, \text{mm}^2 = 0.0000005 \, \text{m}^2 \)
• \( L = 2 \, \text{m} \)

Calculation:

\[\rho = \frac{R \cdot A}{L} = \frac{10 \cdot 0.0000005}{2} = 2.5 \times 10^{-6} \, \Omega \cdot \text{m}\]

Result: \( \rho = 2.5 \times 10^{-6} \, \Omega \cdot \text{m} \)

Example 2

Given:

• \( R = 5 \, \text{k} \Omega = 5000 \, \Omega \)
• \( A = 1 \, \text{cm}^2 = 0.0001 \, \text{m}^2 \)
• \( L = 50 \, \text{cm} = 0.5 \, \text{m} \)

Calculation:

\[\rho = \frac{R \cdot A}{L} = \frac{5000 \cdot 0.0001}{0.5} = 1 \, \Omega \cdot \text{m}\]

Result: \( \rho = 1 \, \Omega \cdot \text{m} \)

Typical Resistivity Values

• Silver: \( 1.59 \times 10^{-8} \, \Omega \cdot \text{m} \)
• Copper: \( 1.68 \times 10^{-8} \, \Omega \cdot \text{m} \)
• Aluminum: \( 2.82 \times 10^{-8} \, \Omega \cdot \text{m} \)
• Iron: \( 9.71 \times 10^{-8} \, \Omega \cdot \text{m} \)
• Silicon: \( 640 \, \Omega \cdot \text{m} \)